Main Research
Click
here for my research with undergraduates!
I work in the field of algebraic combinatorics with a focus on combinatorial models
of representations of Lie algebras. This pertains mainly to the use of combinatorial
structures to encode complex algebraic objects and then make computations and
conclusions using combinatorial techniques. The idea is that the combinatorial models
are simpler to manipulate than the original algebraic object, but maintain all of the
structure.
Main Combinatorial Object
(Kashiwara Crystals)
Crystal bases were independently discovered by Kashiawara and Lusztig in the early
1990s as a means of deciphering nice combinatorial properties of representations
of quantum groups (noncommutative analogs of Lie groups) and are the result of taking
the limit of the quantum groups parameter q to zero. Not only do these crystals have
very nice combinatorial structure, as they can be modeled by colored directed graphs,
but they also encapsulate all of the structure of the corresponding representation.
The edges of these graphs are colored by crystal operators which are related to the
corresponding Chevalley generators.
Here are
some slides
from an undergraduate oriented talk which describe the Tableaux model for a certain type of these crystals in a
purely combinatorial manner.
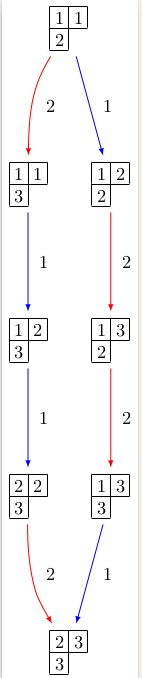 And example of a crystal graph of type A (made in Sage )
B. Salisbury, A. Schultze, and P. Tingley. Combinatorial descriptions of the
crystal structure on certain PBW bases. Transform. Groups, 23: 501-525, 2018.
Link
And example of a crystal graph of type A (made in Sage )
B. Salisbury, A. Schultze, and P. Tingley. Combinatorial descriptions of the
crystal structure on certain PBW bases. Transform. Groups, 23: 501-525, 2018.
Link
B. Salisbury, A. Schultze, and P. Tingley. PBW bases and
marginally large tableaux in type D. J. Comb., 9:535-551, 2018.
Link
C. Lecouvey, C. Lenart, A. Schultze. Towards a Combinatorial Model for q-weight
Multiplicities of Simple Lie Algebras. ArXiv 2110.15394.
Extended Abstract Link
C. Briggs, C. Lenart, A. Schultze.
On Combinatorial Models for Affine Crystals. ArXiv:2109.12199.
Extended Abstract Link
